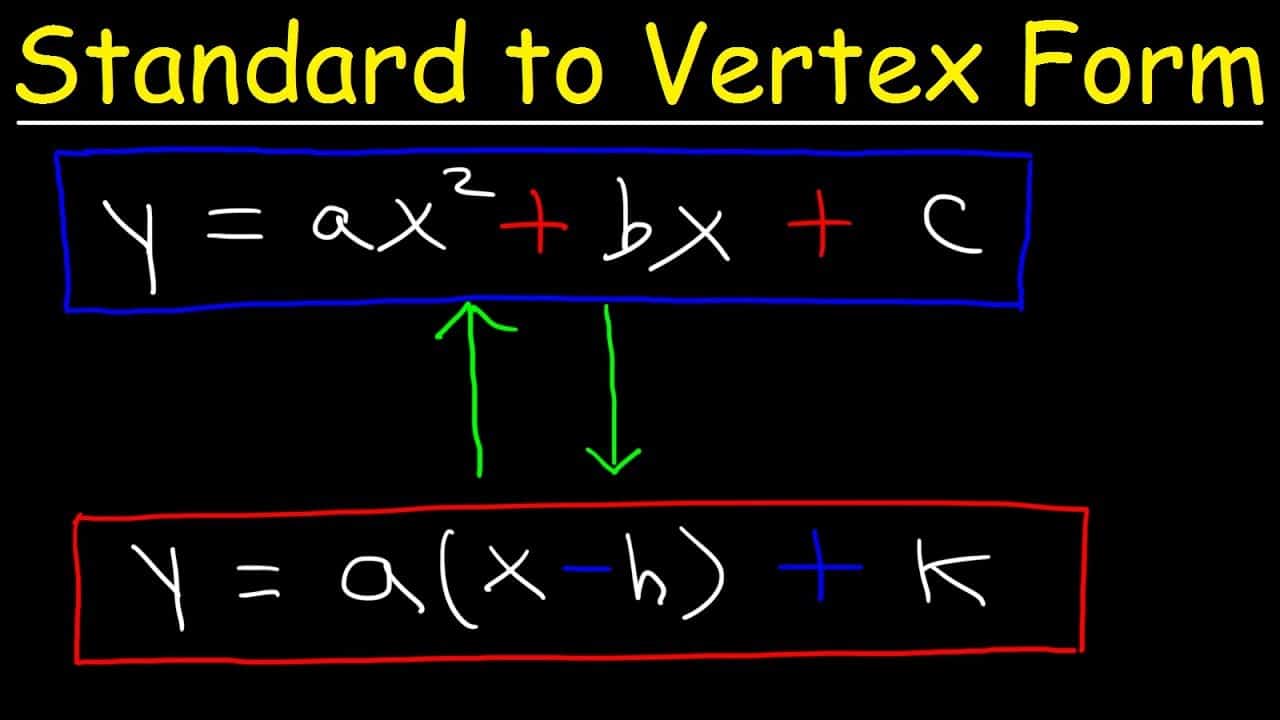
In math, the vertex formula is used to determine the location of vertices of a parabolic curve when the graph intersects the symmetrical axis. In most cases, a vertex point is shown by (h, k). The conventional formula for a parabola is y=ax2+bx+c. If the x2 coefficient is +ve, the apex must be located near the base of that U-shaped curvature. If the x2 coefficient is -ve, the apex must be located at the highest point of the U-shaped curvature.
Definition of a Parabola
A parabola is a group of spots all of which are the same length from one focus (a stationary position) as well as a directrix (a stationary line). Whenever one plots any quadratic function in a graph, the “u” shape which is formed is the parabola.
The parabolic curve exposes the right, left, up, or down based upon the coefficients of a given equation.
The Symmetry Axis of a Parabola
Let’s go through this prior to identifying the apex of a parabolic curve.
Notice that all the points in a parabolic curve contain an x as well as a y coordinate that fits the quadratic equation.
A vertical line that passes in via the apex of a parabolic curve is known as the symmetric axis. The apex of a parabolic curve is thus the greatest or smallest position upon that quadratic equation graph.
Keep in mind that each and every quadratic equation has a basic form.
y=ax2+bx+c.
The formula of a parabolic curve’s central axis would be as follows:
x=-b/2a
Vertex Form of Parabola
The apex of one parabolic curve is the place at which the parabola’s axis of symmetry intersects. If its x2 variable’s coefficient is +ve, the apex would be the deepest place on the given graph, at the base of a “U “-structure. If it’s x2 the term’s coefficient is -ve, the apex becomes the topmost place on the given graph, at the summit of the ” U “-structure.
The conventional formula of a parabolic curve is given as y=ax2+bx+c.
As a result, y = a(x-h)2 + k would be the vertex formula of a parabolic curve.
Let’s first go through the vertex equation in more depth.
Vertex Formula
To get the vertices of a parabolic curve, we will be using the vertex formula. Following are the main two methods for determining the apex of a parabolic curve.
Vertex, (h, k) = (-b/2a, -D/4a)
Here “D” denotes the discriminant, and D = b2 – 4ac.
The vertex coordinates are “h” & “k.”
This equation may alternatively be expressed in the following form:
Vertex = (h,k) = (-b/2a,c – b2 /4a)
The following is another way for determining the apex of a parabolic curve:
A x-coordinate of a vertex, (i.e. h), is known to be -b/2a.
Therefore, we may retrieve the y-coordinate of a vertex by substituting the x-coordinate quantity in the supplied simple form of the formula of parabola y=ax2+bx+c.
Online Math Classes
In general, we notice that most pupils avoid the topic of mathematics. One of the key causes for such fleeing or dread of the issue is a lack of a solid foundation or ground. The Cuemath service is an online live education platform that is created with the aid of subject-matter experts. Their online math classes place a strong emphasis on student idea clarity and tie theoretical doubts to real-world situations so that students may readily learn and grasp numerous concepts. Cuemath’s website is distinctive and distinct from others. It distinguishes itself from the rest by adhering to five distinct fundamentals: end aim, instructional approach, class participation, subject focus, & practice style. Attend Cuemath’s online math classes to experience an interactive way of learning.